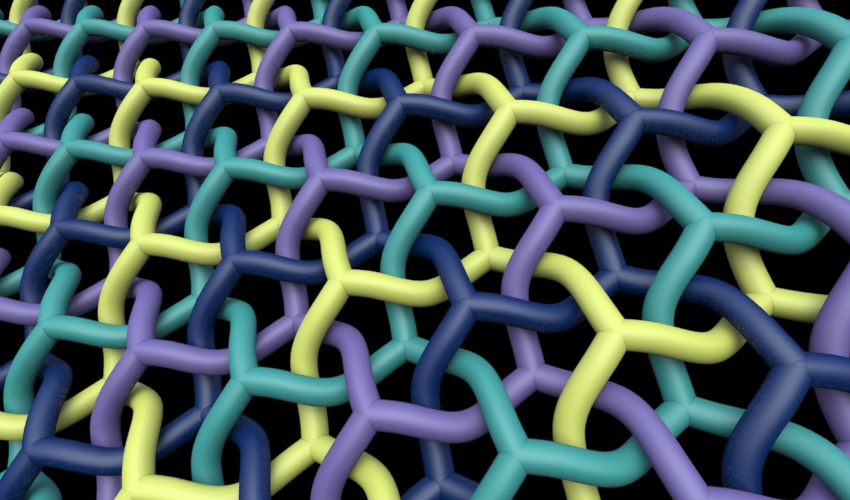
Framework materials composed of rigid bars and joints and their deformations provide a compelling relation between materials science and algebraic geometry. Physical distance constraints within the material transform into quadratic polynomial constraints via the Euclidean distance between two points, enabling associated numerical strategies for finding feasible configurations and deformation pathways. When adding elastic elements, the resulting material is called a tensegrity framework and can be used to model curvilinear cylinder packings. In this paper, we build the necessary geometric formulations to robustly transform rod packings to tensegrity frameworks, leading to a nonlinear optimization problem with polynomial constraints. Since the constraints are smooth almost everywhere, techniques from Riemannian optimization become admissible, allowing us to provide numerical strategies for exploring the deformative behavior of two exemplary 3-periodic cylinder packings. As a result of this investigation, we are able to show that the structures are auxetic by multiple definitions, intuitively meaning that when expanded in a fixed direction, these materials also expand in their lateral directions.